Mixed Numbers and Improper Fractions

Mixed Numbers

A mixed number is a combination of a whole number and a fraction. For example, if you have two whole apples and one half apple, you could describe this as 2 + 1/2 apples, or 2 1/2 apples.


Writing Mixed Numbers as Fractions

This mixed number can also be expressed as a fraction. Each whole apple contains two half apples. Your two whole apples are also four half apples. Four half apples plus one half apple is five half apples. So you have 5/2 apples.

To put this another way: to turn a mixed number into a fraction, multiply the whole number by the denominator (the bottom part), and add the result to the numerator (the top part).

2 1/2 = ?
  Multiply the whole number by the denominator.
    The whole number is 2.
    The denominator is 2.
    2 x 2 = 4.
  Add the result to the numerator:
    The numerator is 1.
    4 + 1 = 5
  The numerator is 5. The denominator remains 2.
2 1/2 = 5/2

More Examples:







Proper and Improper Fractions

A fraction in which the numerator is smaller than the denominator, like 1/3 or 2/5 is called a proper fraction. A fraction in which the numerator is larger than or equal to the denominator, like 5/2, 17/3, or 6/6 is called an improper fraction. (To put it another way, a fraction with a value less than 1 is a proper fraction. A fraction with a value greater than or equal to 1 is an improper fraction.)

As shown above, mixed numbers can be written as improper fractions. Similarly, improper fractions can be written as mixed numbers.


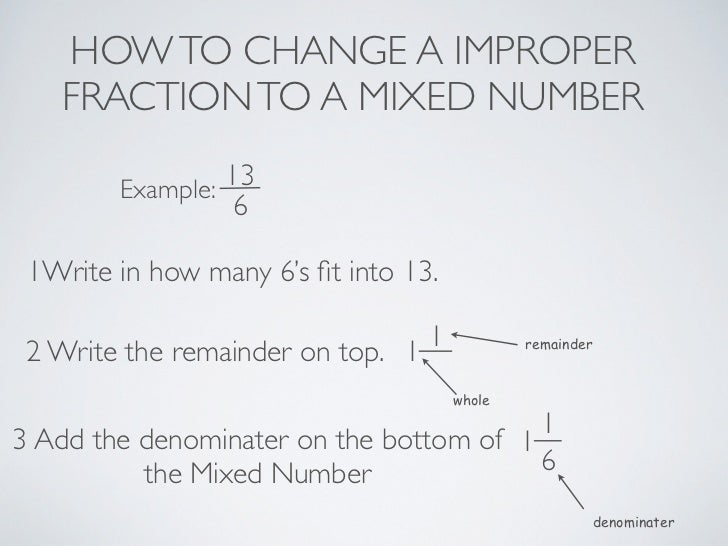
Writing Improper Fractions as Mixed Numbers

To write an improper fraction as a mixed number, divide the numerator (top part) by the denominator (bottom part). The quotient is the whole number, and the remainder is the numerator.

How would you express 17/4 as a mixed number?
  Divide the numerator by the denominator:
  17 ÷ 4 = 4, with a remainder of 1
  The quotient, 4, is the whole number. The remainder, 1, is the numerator. The denominator remains 4.
17/4 = 4 1/4

More Examples:









Here is a comparison of the conversions side by side.




Homework this week will have your kiddo practicing this concept. It will be due on Monday December 12th. Please let me know if you have any questions!